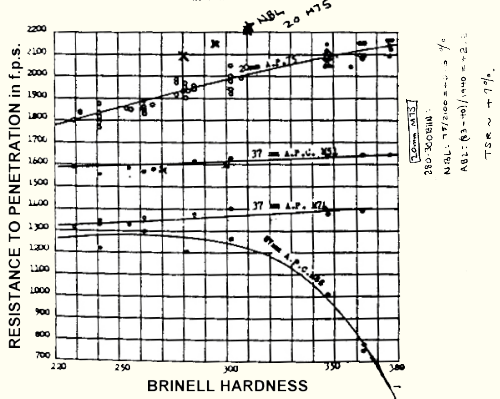
An increase in the hardness of a given thickness of homogeneous armour may result in an increase or in a decrease or in no change at all in resistance to penetration, depending upon the ratio of the thickness of the armour to the calibre of the projectile concerned. Where under-matching projectiles (those whose calibre is less than the thickness of the armour) are concerned resistance to penetration at normal impact increases as hardness increases; where overmatching projectiles (those whose calibre is greater than the thickness of the armour) are concerned, resistance to penetration at normal impact decreases as hardness increases; and where matching projectiles (those whose calibre equals the thickness of the armour) are concerned, little change in resistance to penetration at normal impact occurs over a considerable range in hardness.
As the ratio of thickness to calibre approaches closer to 1:1, the effect of hardness upon resistance to penetration becomes less, and as that ratio either increases or decreases from 1:1, the effect becomes greater. The relationships are illustrated in Fig VII-4 [below].
Fig VII-4: The Effect Of Hardness Upon Resistance To Penetration With Undermatching, Matching, And Overmatching Projectiles.
1½” Rolled Homogeneous Armor Tested with
20mm A.P. M75,
37mm A.P. M74 & A.P.C. M51, and
57mm A.P.C. M86,
projectiles at NORMAL IMPACT.
This information above was supplied to me by Jim O’Neil (Arizona, USA), the original source was either Nathan Okun or Lorrin Bird. I’ve added a couple of quick comments below in the Notes section.
Notes
As can be seen, the equally matched projectiles (i.e. 37mm vs 1½” Plate) have a small but steady increase. For the 37mm with A.P. M74 to penetrate 1½” of typical British or U.S. Armour Plate (with a Brinell Hardness of about 245) requires a velocity of about 1,330 fps, but to penetrate 1½” of German Plate (about 300 BHN – Brinell Hardness) requires a velocity of about 1,370 fps. Note how the 37mm Capped round (A.P.C.) requires considerably higher velocity than normal A.P. round (however against Face Hardened Armour the relationship changes considerably).
However once we look at the undermatched projectiles (i.e. 20mm vs 1½” Plate) we see it takes a velocity of about 1,850 fps to penetrate the Brirish/U.S. Armour (245 BHN) but requires 1,990 fps to penetrate the equivalent of German Armour (300 BHN) – a significant difference. Note that the 20mm round is undermatched by a ratio of 1:1.905.
Finally, for overmatched projectiles (i.e. 57mm vs 1½” Plate) we see it takes a velocity of about 1,290 fps to penetrate the equivalent of British/U.S. Armour (245 BHN), but against the “harder” German Armour (300 BHN) the velocity required is only about 1,240 fps! How many wargaming rules include that?! The 57mm round is overmatched by a ratio of 1.496:1.